
In the current post, I will show that the "unknowability hypothesis" - which is the basic epistemological assumption of Barth - is logically vacuous, and defies the Law of Non-contradiction. We are reminded that any proposition that contravenes the Aristotelian Law cannot be true.
Suppose, for a reductio, we accept the proposition that p = "God is unknowable" is true. In fact, let us agree with the Barthians concerning the unknowability of God that p = "God is X" is true, where X is an attribute of the noumenal God which is unknowable, and therefore, not known and can never be known. We agree, for argument sake, that we are ignorant of some truth concerning God (i.e. that p) which can never be known via Scripture (or by any other means for that matter). And so we suppose that p is true but not known to be true; then (p ∧ ¬Kp) is true.
Therefore, in accordance with the "unknowability of God" hypothesis, Barthians claim that (p ∧ ¬Kp) is true. (2) Furthermore, they know that (p ∧ ¬Kp) is true, that is, ◊K(p ∧ ¬Kp). (3) Now, this is extremely difficult for any logical mind to receive. How can we know that p ∧ ¬Kp? If knowing a conjunction entails knowing the conjuncts, then K(p ∧ ¬Kp) entails Kp and K¬Kp. Now knowledge entails truth (or more accurately, justified true belief), so K¬Kp entails ¬Kp, which is a contradiction for Kp. So, by reductio ad absurdum, it is not possible that K(p ∧ ¬Kp). We have hereby refuted the "unknowability of God" hypothesis of Neo-Orthodoxy.
To further elucidate the problem of the unknowability hypothesis, we understand that the basic premise of Neo-Orthodox epistemology is K(p ∧ ¬Kp). If p = "God is X," and X represents any attribute which is unknowable of God, then the Barthian's denial of the knowledge of X would paradoxically mandate the knowledge of X. Let us say that p = "God is unknowable." We have seen that Barthians insist that p ∧ ¬Kp is true. Knowledge of p ∧ ¬Kp would entail Kp and K¬Kp i.e. knowing that "God is unknowable," and at the same time, knowing that "God is unknowable" is unknowable. This is a contradiction of the Law of Non-contradiction. In other words, if the Barthian claims that they do not know that p, they must also admit that they know that p.
The onus is therefore on those who insist that "God is unknowable" to show that their epistemological presuppositions are logically viable and coherent.
Notes
1. The "Unknowability of God" hypothesis is basically the belief that God, who belongs to the noumena, is unknowable to the mind of man. This must be distinguished from the Reformed understanding of "Finitum non Capax Infiniti," or "the finite is unable to contain the infinite." The Latin phrase should be understood within the context of the Incarnation of Christ. As Frame had aptly commented, "In the incarnation, Calvin argued, God was manifested in human flesh. However, because nothing finite can completely contain the infinite (finitum non capax infiniti), Christ is also active outside the flesh of Jesus. No less than Luther, Calvin insisted that God wills to be known only in Christ. But he did not believe this meant that God is revealed only in the incarnation; Christ, the eternal Word, also operates outside the work of Jesus. Lutheran critics of Calvin's Christology called this the extra calvinisticum." (John Frame, "Incarnation," in The Westminster Handbook of Reformed Theology, ed. Donald K. McKim (Louisville, KY: Westminster John Knox Press, 2001), 120.)
2. Where p = "God is X," including the proposition "God is unknowable."
3. For those new to modal logic, the basic unary modal operators are usually written □(or L) for Necessarily and ◊(or M) for Possibly. In modal logic, each can be expressed by the other and negation, that is:
Re: A clarification for those who are perplexed
I apologize for the use of symbolic logic which may be confusing for some, but it allows some of us to see the picture in a neat, mathematical way.
My point in this post, and the previous one as well, is this: we cannot teach that God is unknowable. Firstly, we cannot know that God is unknowable since this entails a logical contradiction. Secondly, if we do not know that God is unknowable i.e. p ∧ ¬Kp, we should abstain from teaching such a doctrine in theology classes. If you do not know that Tom is unsaved, would you teach others that Tom is unsaved with any certainty?
If God is unknowable (and Barth insists that He cannot be known even through Special Revelation), he has attributes that cannot be known i.e. p = God is X, where X is any unknowable attribute of God. Therefore, with or without the agreement of Barth, if he teaches that "God is unknowable," that proposition can be expressed as p ∧ ¬Kp. Since Barth teaches this in his Church Dogmatics, he must be fairly dogmatic (pun intended) concerning this.
But as we have seen above (in the two posts), to say that God is unknowable (and to say that you know that God is unknowable) is to say ◊K(p ∧ ¬Kp), and this entails Kp and K¬Kp. If you were to look at this expression carefully, you would notice that, to claim knowledge in the proposition that you cannot know p i.e. (K¬Kp), you must also claim Kp. To put this simply, to say that you know that you cannot know that p = God is X, you must paradoxically know that p. But this makes sense. To say, for example, that you know that you cannot know that God is X, you must know what God is X is in order to know that you cannot know that God is X! Contained within the proposition that "you know that you cannot know that God is X" is "God is X," and that logically entails knowledge of "God is X." Because without knowledge of "God is X," we cannot even claim knowledge that "we cannot know that God is X."
But the problem here is: p ∧ ¬Kp is possibly true. Then we can simply say that it is possible that God has certain attributes that we can never know, but we cannot know this for sure (i.e. it is not possible that we know this). In other words, we cannot say that God is unknowable. For surely God is knowable through Scripture, for scriptural revelation is propositional and clear. There may be attributes of God that we do not know and will never know this side of eternity, but we do not know this, and we cannot teach this as part of our theology proper.

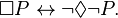
Hmmm, is it good to know more about modal logic? Or how about Predicate logic? My elementary learning of logic was only limited to Aristotelian and propositional logic forms, and of course truth tables.
ReplyDeleteAnyway I agree with you, but is it necessary to include the necessary/possibility argumentation in order to create a reductio ad absurdum for Neo-Orthodoxy?
Dear Daniel,
ReplyDeleteThanks for your comments!
The "modifiers" here are used as epistemic modalities.
I would have to state that K(p ∧ ¬Kp) is only possibly (and not necessarily) true, even for the Barthians. We can all agree that the proposition is not necessarily false. It is not required for the reductio, but is in place as an anticipation of certain responses.
I do not want to put the proposition in a manner that the Barthians have never admitted i.e. that K(p ∧ ¬Kp) is a necessary truth; they would claim that even such knowledge is paradoxical i.e. it is "possible" that they would claim K(p ∧ ¬Kp) ∧ ¬K(p ∧ ¬Kp) is a possibility. :)
More importantly, Axiom B states that:
p > LMp or
p→□◊p
So the fair thing to do is to represent the possibility.
But if K(p ∧ ¬Kp) as a possible truth is shown to be illogical, impossible and untrue, then it cannot be a necessary truth.
Yours truly,
Vincent
My head got dizzy reading this, so I read it again. While we may never be able to know completely or understand completely God, I use to think God was un-knowable.
ReplyDeleteRather, He was quite distant from man and then He changed my mind.
Vince, you certainly bring a smile to my face while I read on your blog, but I have to admit... you make me think real hard on some of the things you write about...
God bless you and keep you close knitted to Him.
{by the way, although I was not able to truly understand the
K(p^~Kp)
I still enjoyed reading it.
Hi Brother,
ReplyDeleteThanks for your insights.
The issue at hand is not whether Man can have complete knowledge of God. No doubt we creatures cannot know everything about God, at least on this side of eternity. In my previous post on "the unknown God," I have emphasized that we have only an "apprehensive" knowledge of God, and not a "comprehensive" knowledge of the Divine.
But there are some who insist that, even those things that we do know i.e. the fundamentals of Christianity, we cannot know that we know. In other words, these theologians want evangelicals to repudiate any certainty in our knowledge of God. And this underlies their attitude towards the Bible - "we can never know anything about God through the Bible. We think we know, but we do not know." They encourage us to adopt "epistemic humility," which is basically, "don't be too dogmatic about any thing you say!"
Remember the familiar aphorism, "This is your interpretation, and that's mine. We can't know for sure which is correct"?
Peace be with the moderator, as well as those able to read this message.(if not censored)
ReplyDeleteThe time has come, the harvest is ripe.
Make sure to share this with fellow believers.
The Faithful Witness
Hi Vincent,
ReplyDeleteThe answer is still - "I am the LORD thy God..."
Sincerely,
Jenson
Hi Vincent,
ReplyDeleteI followed the argument with some difficulties (then I realized that if I ignored the notes on modal temporal logic, the post made more sense..). So it could be that I am still confused as to the thrust of the argument. So I am trying to reproduced it in my own notation.
P and not(K(P)) -
P is true and P is not knowable is true. This is assumption A.
K(P and not (K(P)) -
The knowledge of assumption A to be true. This is proposition B.
K(P) and K(not(K(P)) -
The knowledge that P is true and knowledge that knowledge of P not possible. This is proposition C which is an expansion of proposition B.
Thus, we have arrived at a contradiction since both K(P) and K(not(K(P)) have to be true for proposition C to stand. Thus, the assumption A is false.
However, I am not quite at ease with this formulation (not quite tight enough, it seems to me). Given my limited exposure to neo-orthodoxy, I am quite uncertain as to whether Barth would agree that the assertion of the unknowability of God is to be formulated as assumption A above.
Furthremore, even if we do grant that the formulation of assumption A is accurate enough. It seems to me that when one assumes A as a pre-supposition (in the pure logical sense), whether one is actually making a further claim that one knows A to be true. It is entirely possible to presume A to be true without knowing it to be true. (Of course, I understand that the issue at stake is whether A is a legitimate pre-supposition, but from the viewpoint of a construction of an argument, it seems to me that your argument fails at this step.) It seems to me that the formulation must be nuanced somewhat for it to be more convincing.
my 2 cents,
Edward
P.S. From a scriptural point of view, it seems that there are many facets to the "knowing" of God. On one hand, Paul speaks of unbelievers as suppressing the knowledge of truth (surely one must know before one can suppress it?). But on the other, he is clear that a work of "re-creation" must takes place in the soul for the gospel to be un-veiled (cf. 2 Cor 4:6). The noetic effects of sin seems to be quite key in this discussion.
Dear Sim Joon,
ReplyDeleteThank you for your input, and for visiting my blog.
You wrote, "It is entirely possible to presume A to be true without knowing it to be true." I agree.
But this is the crux of the problem. Barth is very dogmatic when it comes to this i.e. that God is unknowable. It appears that Barth knows this. :)
Why else would you teach it (as true) if you do not know that it is true?
For example, using your "A",
Let P = God is Unknowable (which is Barth's hypothesis)
That leads to an obvious contradiction. Any further attribute of God that Barth claims to know would add to the logical confusion/contradiction.
The main thrust of this post is actually in my previous post (i.e. that you cannot insist that God is unknowable, and yet claim to know anything about God). This brief argument is only an addendum to it.
"Given my limited exposure to neo-orthodoxy, I am quite uncertain as to whether Barth would agree that the assertion of the unknowability of God is to be formulated as assumption A above."
Why not?